Joseph Fourier
(1768 - 1830)

If f is 2Pi-periodic and differentiable by pieces then for
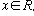
with

which can be written in complex numbers, and for a T-periodic function , :

Aspects of his life

Fourier has a special place in the maths world, and also in the world of converging series. Born in 1768, Fourier was obsessed by the study of heat. Although his lodgings in Grenoble were overheated, which bothered his visitors, he was cramped in heavy clothes. But to study these phenomena, he finalised a method of splitting up periodic signals into sums of more simple signals, particularly sinusoidal ones. Technicians rarely need more than a few signals to reconstruct the required signal with a good approximation. But the mathematician knows the exact division into weaker and weaker signals. If we have a continuous function f, 2 periodic, and derivable, it can be split up as above. The formula stays valid if it is continuous and derivable by intervals, we just need to replace f(x) by (f(x-)+f(x+))/2, where x is a discontinuous point. This theory however was not very welcome at the time. Many famous mathematicians, among them Lagrange, Laplace, Legendre, Biot and Poisson, did not accept this conjecture, and when Fourier exposed it to the academy of Science, Lagrange stood up and declared that he considered it wrong! Because of the overheating, Fourier died from heart failure in 1830 !

Around

Nowadays, to find the numerous formulae in Euler books, for example, we use Fourier's theory. The general formula allows one to calculate the limit of nearly all the series by numerous examples...

Moreover, it is amusing to see that even nowadays, the techniques used to accelerate calculation in the actual algorithms are based on the "transformées de Fourier rapide (TFR)", and allow one to reach billions of digits calculated on computers... Mr Fourier is decidedly very helpful !

Demonstration

Let's not worry about the demonstration of the formula called "Dirichet's theorem", quite long and without any direct link with . However let's detail it a little bit:

Let f be a function of R in C, 2Pi-periodic and continuous in pieces in Moreover, let x be a point where f is differentiable on the right and on the left. Now, let where . Thanks to a classical equality , the Chasles relation and the variable change , we now have the following result . According to Dirichlet, S_n(x) converges towards .

Applications

It is here that Fourier's theory becomes useful for us ! One just needs to choose a function verifying the theorem's conditions and to calculate its "Fourier's coefficients". Then one chooses a particular value of x :

1) If f is the 2Pi-periodic function for , we find , the other coefficients being nul (bn=0 anyway because f is a symmetric function, f(x)=f(-x)). Thus, ... in particular, when x=0, we get , which is just one of the several Euler's formulas !

2) If f(x)=x² for , we obtain the formula .

In particular, with x=Pi, we get .

Also, with x=Pi/2, we get . Fun, isn't it ??

3) Leibniz's formula :

If f(x)=1 when 0<x<Pi and f(x)=-1 when -Pi<x<0, we get for x not equal to k.Pi the formula . With x=Pi/2, our eyes out on stalks discover that !

4) The best of it !

If f(x)=cos(zx) for and , after computing the Fourier's coefficients we obtain that is, with x=0:

or with x=Pi,

(again, we recognize one of Euler's formula). Now, if we differentiate three times each part of the previous formula (we're allowed to do so since the sum uniformly converges for z in ]0,1[, we obtain . Taking z=1/2 now gives that we can transform: with . Brilliant !

Attempts

Look back at Euler for the attempts at speed of convergence of the series given by Fourier's formulae.